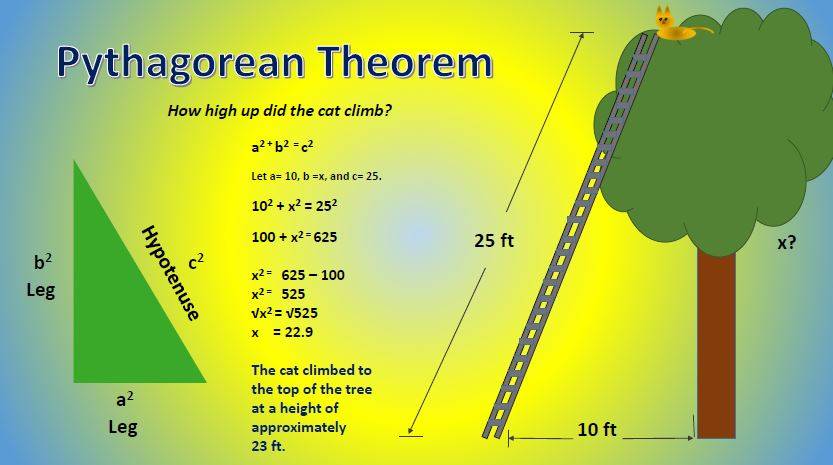
THE GREAT THEOREM OF PYTHAGORAS
What is Pythagoras theorem, what is its proof and applications.
Answer:
Pythagoras Theorem: It is a theorem that describes the relation among the sides of a right-angled triangle. Pythagoras Theorem is commonly used to find the length of an unknown side in a right-angled triangle. The sides of a right-angled triangle are usually described as the base, perpendicular, and hypotenuse. Example is given below:
The Pythagoras Theorem states that in a right-angled triangle the sum of the square of the base and square of the perpendicular is equal to the square of the hypotenuse.
Pythagoras Theorem Formula
From the right angled triangle above, the Pythagoras theorem formula is given by the equation;
where
, YZ – base, XY – perpendicular and XZ - hypotenuse
It must be noted that Pythagoras Theorem is applicable for only the right-angled triangle.
Pythagoras Theorem Proof
Considering ∆ XYZ with ∠XYZ = 90° as shown above and to prove that
From the diagram above,
in ∆XOY and ∆XYZ, is a common angle
∠XOY = ∠XYZ = 90°
Therefore, ∆ XOY and ∆ XYZ are similar triangles
Also, in ∆YOZ and ∆XYZ is a common angle
And ∠YOZ = ∠XYZ = 90°
Therefore, ∆ YOZ and ∆ XYZ are similar triangles
From (1) and (2) we get,
, hence proved.
Application of Pythagoras Theorem
The Pythagorean Theorem has a wide range of applications in everyday life. They include the following
1. Pythagoras Theorem is commonly used to find the unknown side of a right-angled triangle.
2. Pythagoras Theorem is used in trigonometry to find the trigonometric ratios.
3. Pythagoras Theorem used in security cameras for face recognition.
4. The technique of Pythagoras Theorem is used by architects at the engineering and construction fields.
5. The Pythagoras Theorem is applied in surveying mountains.
6. It is useful in navigation to find the shortest route.